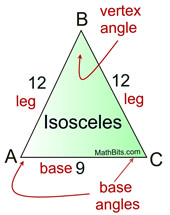
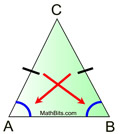
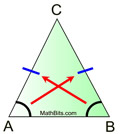
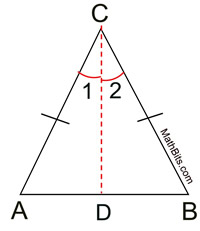
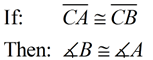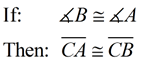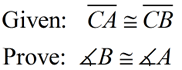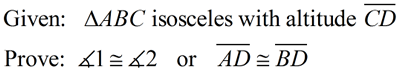Proof:
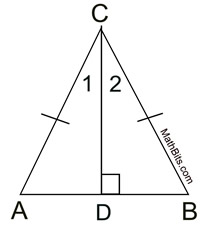
• Construct an auxiliary line through point C bisecting ∠C. An angle has a unique angle bisector. Label the intersection with the base as D. • m∠ACD = m∠BCD because an angle bisector forms two congruent angles which have equal measure. • Under a reflection in • Since m∠ACD = m∠BCD and reflections preserve angle measure, the image of ∠ACD will be the same measure as ∠BCD. • Since these angles are equal in measure, the reflection of ray • The reflection of • The reflection of • The reflection of A is B since reflections preserve length and the segments share point C. • The reflections of • The reflection of ∠CAB will have the same measure as ∠CBA since reflections preserve angle measure. We have established that the rays forming these angles coincide under a reflection. • QED
Topical Outline | Geometry Outline | MathBitsNotebook.com | MathBits' Teacher Resources
|