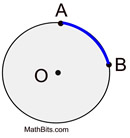
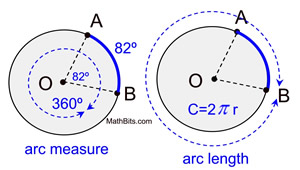
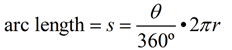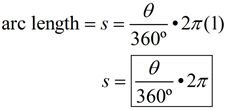An arc of a circle is a "portion" of the circumference of the circle.
The length of an arc is simply the length of its "portion" of the circumference. The circumference itself can be considered a full circle arc length. |
 |
|
Arc Measure: In a circle, the degree measure of an arc is equal to the measure of the central angle that intercepts the arc. |
|
|
Arc Length: In a circle, the length of an arc is a portion of the circumference.
The letter "s" is used to represent arc length. |
|
|
Consider the following proportion:
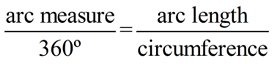 |
If we solve the proportion for arc length (by cross multiplying), and replace "arc measure"
with its equivalent "central angle", we can establish the formula:
Notice that arc length is a fractional part of the circumference.
For example, an arc measure of 60º is one-sixth of the circle (360º),
so the length of that arc will be one-sixth of the circumference of the circle.
Stated as a theorem, we have:
|
If an arc of a circle of radius r has measure n, then the length of the arc is |
|
|

In circle O, the radius is 8 inches and minor arc 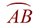 is intercepted by a central angle of 110 degrees. Find the length of minor arc is intercepted by a central angle of 110 degrees. Find the length of minor arc 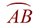 to the nearest integer. to the nearest integer.
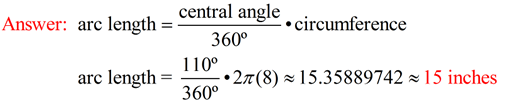

Justify why: The length of the arc intercepted by a central angle is proportional to the radius. |
The diagram at the right shows two circles with the same center (concentric circles). It has already been shown that concentric circles are similar under a dilation transformation.
The ratio of similitude of the smaller circle to the larger circle is:
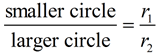 |
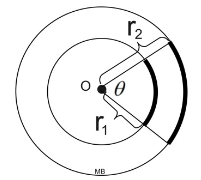 |
| The same dilation that mapped the smaller circle onto the larger circle will also map the slice (sector) of the smaller circle with an arc length of s1 onto the slice (sector) of the larger circle with an arc length of s2. When the radius gets dilated by a scale factor, the arc length is also dilated by that same scale factor. |
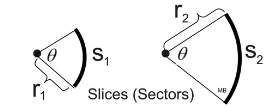 As long as the central angles are the same, the slices (sectors) will be similar. |
Since corresponding parts of similar figures are in proportion, 
An equivalent proportion can be written as 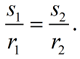 This proportion shows that the ratio of the arc length intercepted by a central angle to the radius of the circle will always yield the same (constant) ratio. This proportion shows that the ratio of the arc length intercepted by a central angle to the radius of the circle will always yield the same (constant) ratio.
In relation to the two arc length formulas seen on this page, both show that arc length, s, is expressed as "some value" times the radius, r. The arc length is proportional to the radius.
When the angle θ is in degrees:
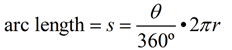
|
Setting r = 1 shows the constant of proportionality.

NOTE: The re-posting of materials (in part or whole) from this site to the Internet
is copyright violation
and is not considered "fair use" for educators. Please read the "Terms of Use". |
|


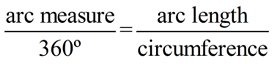
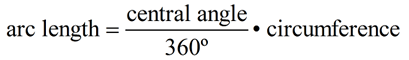


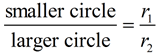

 As long as the central angles are the same, the slices (sectors) will be similar.
As long as the central angles are the same, the slices (sectors) will be similar. 
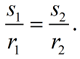 This proportion shows that the ratio of the arc length intercepted by a central angle to the radius of the circle will always yield the same (constant) ratio.
This proportion shows that the ratio of the arc length intercepted by a central angle to the radius of the circle will always yield the same (constant) ratio.